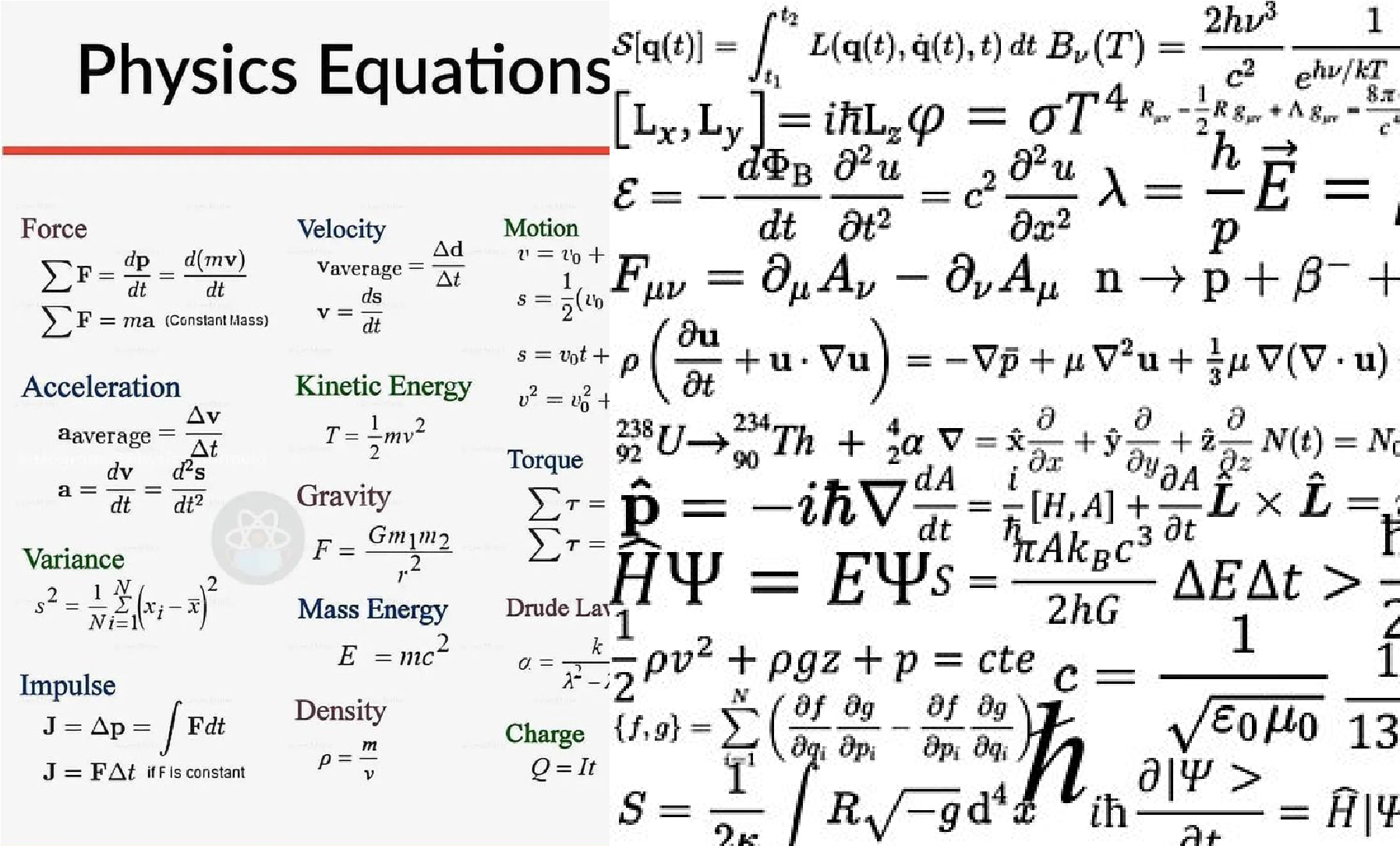
Physics is often described as the study of the fundamental forces and principles that govern the universe. At the heart of this field lies a powerful tool: physics equations. These mathematical expressions allow scientists to describe and predict the behavior of physical systems, from the smallest particles to the largest galaxies. Understanding these equations is key to unlocking the mysteries of the natural world. In this article, we will explore some of the most important physics equations, their significance, and how they are used to model and understand the universe around us.
The Role of Equations in Physics
Physics equations serve as a universal language that translates complex physical phenomena into a form that can be analyzed and understood. They are the backbone of theoretical and experimental physics, providing a framework for scientists to test hypotheses, develop new theories, and make predictions about the behavior of matter and energy.
Equations in physics often involve quantities such as force, mass, velocity, energy, and momentum, which are interrelated in ways that describe how objects move, interact, and change over time. By manipulating these equations, physicists can gain insights into everything from the motion of planets to the behavior of subatomic particles.
One of the key aspects of physics equations is their ability to simplify the vast complexity of the natural world into manageable and understandable models. These models, though sometimes abstract, are powerful tools for making sense of observations and guiding further exploration and discovery.
Newton’s Laws of Motion
One of the most fundamental sets of equations in physics is Newton’s Laws of Motion, which describe the relationship between a body and the forces acting upon it, and the body’s response to those forces. These laws, formulated by Sir Isaac Newton in the 17th century, laid the groundwork for classical mechanics.
First Law (Law of Inertia):
F=0⇒v = constantF = 0 \Rightarrow \text{v = constant}F=0⇒v = constant
This law states that an object at rest will remain at rest, and an object in motion will continue in motion with a constant velocity, unless acted upon by a net external force. This concept of inertia is fundamental to understanding how forces affect motion.
Second Law (Law of Acceleration):
F=maF = maF=ma
This equation expresses that the force acting on an object is equal to the mass of that object multiplied by its acceleration. It quantifies how forces cause changes in motion and is crucial for solving problems involving dynamics and motion under the influence of various forces.
Third Law (Action and Reaction):
F12=−F21F_{12} = -F_{21}F12=−F21
Newton’s Third Law states that for every action, there is an equal and opposite reaction. This principle is essential in understanding interactions between objects, such as collisions and the propulsion of rockets.
These equations are foundational to much of classical physics, explaining a wide range of phenomena from the motion of everyday objects to the orbits of planets.
The Universal Law of Gravitation
Another pivotal equation in physics is Newton’s Law of Universal Gravitation, which describes the attractive force between two masses:
F=Gm1m2r2F = G \frac{m_1 m_2}{r^2}F=Gr2m1m2
Where:
- FFF is the gravitational force between two masses,
- GGG is the gravitational constant (6.674×10−11 Nm2/kg26.674 \times 10^{-11} \, \text{Nm}^2/\text{kg}^26.674×10−11Nm2/kg2),
- m1m_1m1 and m2m_2m2 are the masses of the two objects,
- rrr is the distance between the centers of the two masses.
This equation explains how every object in the universe exerts a gravitational pull on every other object. It is the foundation for understanding planetary orbits, the motion of celestial bodies, and even the dynamics of galaxies. The simplicity and universality of this equation underscore the elegance of physics in describing complex systems with concise mathematical expressions.
Einstein’s Theory of Relativity
Albert Einstein’s theory of relativity introduced some of the most famous and profound equations in physics, revolutionizing our understanding of space, time, and energy.
Special Relativity – Mass-Energy Equivalence:
E=mc2E = mc^2E=mc2
This equation, perhaps the most famous in all of physics, states that energy (EEE) is equal to mass (mmm) multiplied by the speed of light squared (c2c^2c2). This equation reveals that mass and energy are interchangeable; they are different forms of the same thing. It has profound implications, particularly in understanding nuclear reactions and the energy produced by stars.
General Relativity – Einstein Field Equations:
Rμν−12Rgμν+Λgμν=8πGc4TμνR_{\mu\nu} – \frac{1}{2}Rg_{\mu\nu} + \Lambda g_{\mu\nu} = \frac{8\pi G}{c^4} T_{\mu\nu}Rμν−21Rgμν+Λgμν=c48πGTμν
These equations describe how matter and energy influence the curvature of spacetime, which we perceive as gravity. General relativity has been confirmed by many experiments and observations, including the bending of light around massive objects (gravitational lensing) and the precise orbits of planets.
Einstein’s equations provide a more complete description of gravity than Newton’s law, particularly in strong gravitational fields or at high speeds, and they are essential for modern astrophysics, including the study of black holes and cosmology.
The Schrödinger Equation
In the realm of quantum mechanics, the Schrödinger equation is a cornerstone for understanding the behavior of particles at the atomic and subatomic levels:
iℏ∂∂tΨ(r,t)=H^Ψ(r,t)i\hbar \frac{\partial}{\partial t} \Psi(\mathbf{r}, t) = \hat{H} \Psi(\mathbf{r}, t)iℏ∂t∂Ψ(r,t)=H^Ψ(r,t)
Where:
- Ψ(r,t)\Psi(\mathbf{r}, t)Ψ(r,t) is the wave function of the system,
- ℏ\hbarℏ is the reduced Planck’s constant,
- H^\hat{H}H^ is the Hamiltonian operator, representing the total energy of the system.
This equation describes how the quantum state of a physical system changes over time. The wave function Ψ\PsiΨ contains all the information about the system’s state, including the probabilities of finding a particle in a particular position or with a particular momentum. The Schrödinger equation is fundamental to quantum mechanics, allowing us to predict the behavior of particles in atoms, molecules, and even larger systems.
The Laws of Thermodynamics
Thermodynamics deals with the relationships between heat, work, and energy. The laws of thermodynamics are expressed through several key equations that describe these relationships.
First Law (Conservation of Energy):
ΔU=Q−W\Delta U = Q – WΔU=Q−W
This equation states that the change in internal energy (ΔU\Delta UΔU) of a system is equal to the heat added to the system (QQQ) minus the work done by the system (WWW). It is a statement of energy conservation and is fundamental to understanding processes like engine cycles and refrigeration.
Second Law (Entropy):
ΔS≥QT\Delta S \geq \frac{Q}{T}ΔS≥TQ
This law states that the entropy (SSS) of an isolated system always increases over time, and it provides a direction to thermodynamic processes, indicating that they are irreversible. This concept of entropy is crucial in understanding the arrow of time and the efficiency of energy conversion.
Third Law:
As temperature approaches absolute zero, the entropy of a perfect crystal approaches a constant minimum. This law implies that it is impossible to reach absolute zero in a finite number of steps.
These laws govern everything from the functioning of engines to the eventual heat death of the universe, making them central to both theoretical and applied physics.
Conclusion
Physics equations are the keys to understanding the universe. From Newton’s laws of motion to Einstein’s theory of relativity and the Schrödinger equation of quantum mechanics, these mathematical expressions allow us to describe, predict, and manipulate the physical world. They are not just abstract concepts but powerful tools that have led to technological advancements and deeper insights into the nature of reality.
Read more: Jason Kelce Wife: Unveiling the Woman Behind the NFL Star